A Cycloid is a Tautochrone

In 1659 Christiaan Huygens answered a question that came to him as he watched a swinging chandelier in a church: What curve has the property that a bead sliding along it under uniform gravity and with no friction will oscillate with a period independent of the amplitude?

The answer turned out to be the cycloid generated by a circle, as illustrated in Figure 1. Several solutions to this problem have been found; Abel’s is particularly remarkable [1].

Presented here is a very short geometrical proof of the tautochronous property of the cycloid. It is based on the fact that \(\bf{v} \perp \bf{r} \), as explained in Figure 1.¹

To prove that the cycloid is a tautochrone, it suffices to show that the arclength distance \(s\) from the bottom of the cycloid behaves as a harmonic oscillator:

\[\begin{equation}
a = −ks,
\end{equation}\]

where \(a = \ddot{s}\), for some constant \(k\). (This idea, which I had learned from Henk Broer, is attributed to Lagrange.) Because \(a = 0\) when \(s = 0\), we just need to verify that

\[\begin{equation}\tag{1}
 da = −k ds.          
\end{equation}\]

But \(da = d(g ~\mathrm{cos}~ \theta) = −g ~\mathrm{sin}~ \theta~ d\theta\). And from Figure 2 we have \(ds = D sin \theta d \theta\). Comparing these expressions for \(da\) and \(ds\) proves (1) with \(k = g/D\). QED

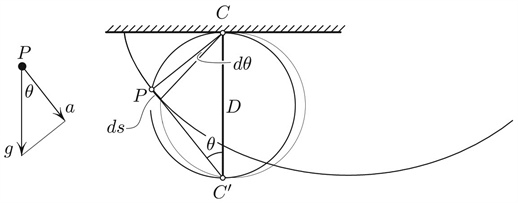
¹ Incidentally, building on this fact, the line of velocity of every point on a rolling wheel (in the ground reference frame) passes through the topmost point of the wheel. A pebble stuck to the tire always aims straight at, or straight away from, the topmost point of the wheel!

References

[1] M. Levi, Classical Mechanics with Calculus of Variations and Optimal Control, AMS, Providence, Rhode Island, 2014.