A Moving Argument

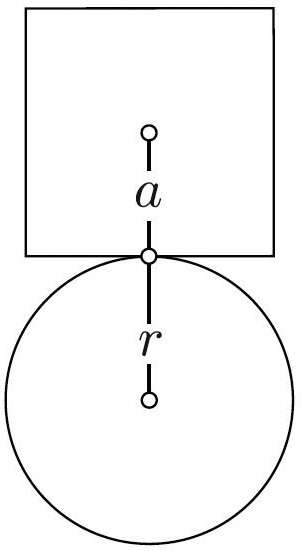
Here is a twist on a well-known problem in mechanics. A cube rests on a sphere, as in Figure 1. The contact is of no-slip kind. What condition on the sizes \(h\) and \(r\) guarantees stability of the equilibrium? 

A Solution by Motion

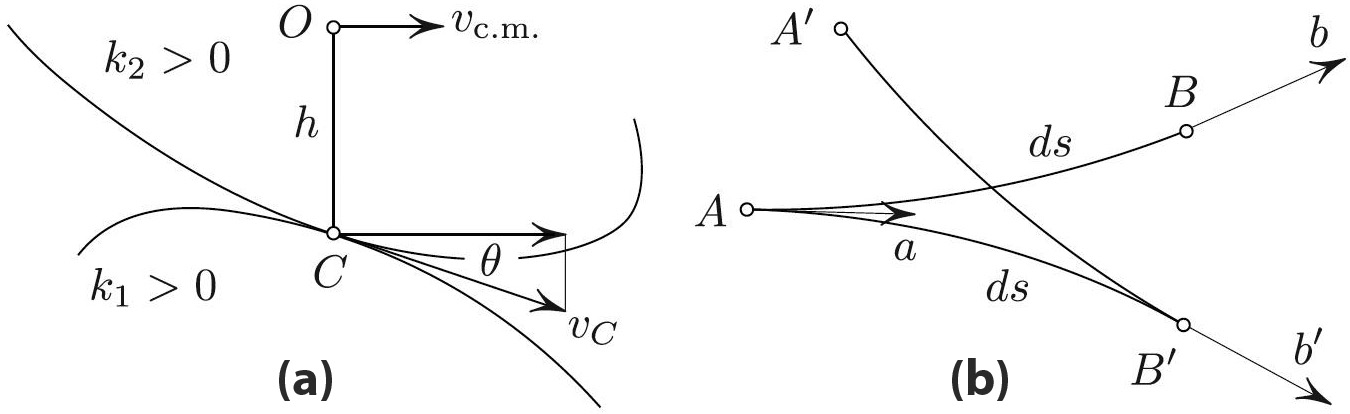
The standard solution involves expressing the cube’s potential energy \(V\) as a function of the tilt angle \(\theta\) and expressing the minimality condition \(V ^{\prime\prime} (0) > 0\) in terms of \(a\) and \(r\). Although this is straightforward, it involves some calculation and is not very instructive. Instead, here is a solution with no calculation. If we roll the cube to the right without sliding (as in Figure 2), both the center of mass and the contact point with the sphere move to the right. The solution then amounts to the observation that the equilibrium is stable if the horizontal velocities satisfy \(v_C> v_{\rm c.m.}\) at the moment the equilibrium is passed.

To translate this criterion into the condition on \(r\) and \(a\), let \(\omega\) be the cube’s angular velocity; \(\omega\) is thus also the angular velocity at which contact \(C\) travels around the circle. We therefore have

\[v_{\rm c.m.} = \omega a, \ \ \ v_C = \omega r . \]

The former is valid at the moment the equilibrium is passed since \(C\) is the instantaneous center of rotation and \(a\) is the distance of the center of mass to \(C\). Substituting these values into our stability criterion \(v_C> v_{\rm c.m.}\) gives

\[\boxed {r> a.}\]

In other words, the equilibrium is stable if and only if the square does not hang over the sides of the circle.

Equivalence of  \(v_C> v_{\rm c.m.}\) With the Minimality of  \(V(0)\) 

According to Figure 2b, the velocity \({\bf v} _{O^\prime} \perp C ^\prime O ^\prime\) and \({\bf v} _{O^\prime}\) is hence sloped upwards so that the potential energy is increasing.

General Shapes

The same idea applies to a general situation of a rock that is resting on a stationary rock (see Figure 3). As we roll the rock, the velocity of the center of mass is horizontal at the moment the equilibrium is passed;¹ the stability criterion is thus \(v_C \cos \theta > v_{\rm c.m.}\). To transform this into a geometrical condition, we note that \(v_{\rm c.m.} = \omega h\) and

\[v_C = \frac{\omega }{k_1+k_2},\tag1\]

where \(k_1, \  k_2\) are the curvatures of the two rocks (positive for convex rocks). Indeed, Figure 3b depicts an infinitesimal segment of the rock that has rolled from position \(AB\) to a new position \(A ^\prime B^\prime\) in time \(dt\) and has rotated (in addition to translation) through the angle \(\omega dt\). Therefore,

\[\angle (b, b ^\prime ) = \omega dt\]

(we count clockwise rotation as positive to avoid dealing with negatives). On the other hand, again treating all angles as positive,

\[\angle (b, b ^\prime ) = \angle (a,b)+\angle(a, b ^\prime ) = k_2 ds+k_1ds.\]

Comparing the last two expressions for \(\angle (b, b ^\prime )\) yields \(\omega dt=(k_1+k_2) ds=(k_1+k_2) v_Cdt\), which amounts to \((1)\).

We conclude that the rock in Figure 3 is stable iff \(v_C \cos \theta > v_{\rm c.m.}\), i.e., if

\[\boxed{\frac{\cos \theta  }{k_1+k_2} >  h .} \tag2\]

For the cube on the sphere in Figure 1, \(k_1=1/r \) and \(k_2=0\), \(h=a\), \(\theta = 0\), and \((2)\) agrees with the result \(r>a\). 

Stability of Tilted Cubes

A cube can rest in equilibrium on any point of the sphere with \(| \theta | < \pi /4\) (see Figure 4a); for the cube to be in equilibrium at a given \(\theta\), the point of contact must be at the distance \(h \tan \theta\) from the midpoint of the side.

Which (if any) of these equilibria are stable? The answer is given by \((2)\). We have \(k_1=1/r\), \(k_2=0\), and \(h = a/ \cos \theta\); the tilted equilibrium in Figure 4 is thus stable precisely if

\[a < r \cos^2(\theta).\tag3\]

For \(\theta\) just under \(\pi /4\), the largest stable cube will be just under \(a =  r/2\).

A Geometrical Criterion

Condition \((3)\) looks nicer when expressed geometrically: the equilibrium is stable iff the cube’s center lies below the arc of the ellipse with the semiaxes \(r\) and \(2r\) (see Figure 4b). If we extend the cube’s base (by a weightless extension) as in Figure 4b, all points on the upper semicircle—not only the ones with \(| \theta | < \pi/4\)—can serve as equilibrium contact points. 

The counterintuitive stability of the small cube in Figure 4b contrasts with the instability of an inverted pendulum. The difference is that the “pivot”—i.e., the contact point—for the cube responds to perturbations so as to create a restoring torque: an inanimate stabilizing mechanism. 

¹This is true because the velocity of \(O\) is orthogonal to the line \(OC\) from the instantaneous center of rotation \(C\), and because \(OC\) is vertical at the moment in question. 

The figures in this article were provided by the author.