Circulation Models Assess the Impacts of Congenital Heart Defects and Hypergravity

When it comes to matters of the heart, control theory offers a helpful way to address many aspects of circulatory biology and medicine. Two contributed presentations at the 2023 SIAM Conference on Control and Its Applications (CT23), which took place this July in Philadelphia, Pa., addressed different aspects of control in blood circulation models. In the first talk, Zan Ahmad of New York University’s Courant Institute of Mathematical Sciences and Johns Hopkins University explained a circulation model that addresses a particular physiology of the heart. Ahmad then delivered a second presentation on behalf of Alanna Kennard (Courant Institute of Mathematical Sciences), who researches the impact of hypergravity on the human circulatory system.

Blood and Oxygen within the Fontan Circulation

To address congenital heart defects in which only one ventricular chamber is functional, clinicians surgically construct a physiology called the Fontan circulation. Blood flows passively to the lungs in this mode of circulation, resulting in a chronically low cardiac output and high systemic venous pressure. Surgeons often create a fenestration, or conduit between the systemic and pulmonary veins, to decrease the total resistance of the system and increase blood flow (see Figure 1) — but this procedure comes with the tradeoff of reduced oxygen saturation.

“First, we’re going to build a model for the fenestrated Fontan circulation,” Ahmad said. “Then, we’re going to adapt the underlying modeling framework to allow for feedback control with a variable heart rate function.” He worked with Charles Puelz (Baylor College of Medicine) and Charles Peskin (Courant Institute of Mathematical Sciences) to implement a pulsatile model and study the impact of fenestration on hemodynamics and blood oxygen content, hoping to determine the optimal size for the conduit [1]. “Fenestration has pros and cons, and we’re going to see if we can optimize this for maximum benefit,” he said.

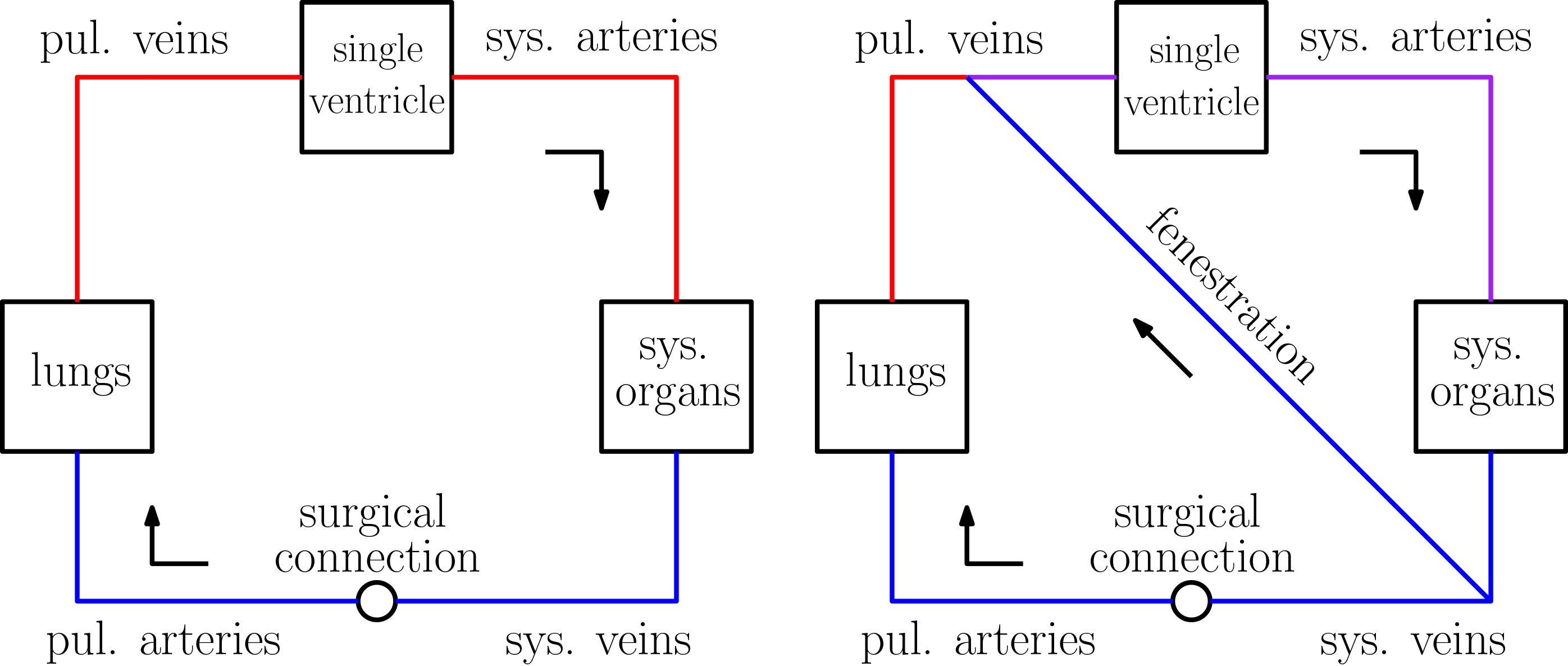
The researchers created a Fontan model that compartmentalizes the circulation into compliance chambers; compliance is a measure of vessel elasticity that is computed as the change in volume over change in pressure. The model maintains a constant compliance for veins and arteries, but allows this parameter to vary over time for ventricles. In an approach that is analogous to electrical circuits, compliance chambers within the model are connected via linear resistor elements with diodes to model valves. “Now, we have equations for blood flow between the different compartments,” Ahmad said.

The model incorporates fenestration as a hole between the systemic and pulmonary veins that has a nonlinear resistance depending on the cross-sectional area. It then uses numerical discretizations to update compartment pressures and oxygen concentrations. To calibrate the model, Ahmad fiddled with parameters to match the model outputs as closely as possible to clinically measured values for real Fontan patients.

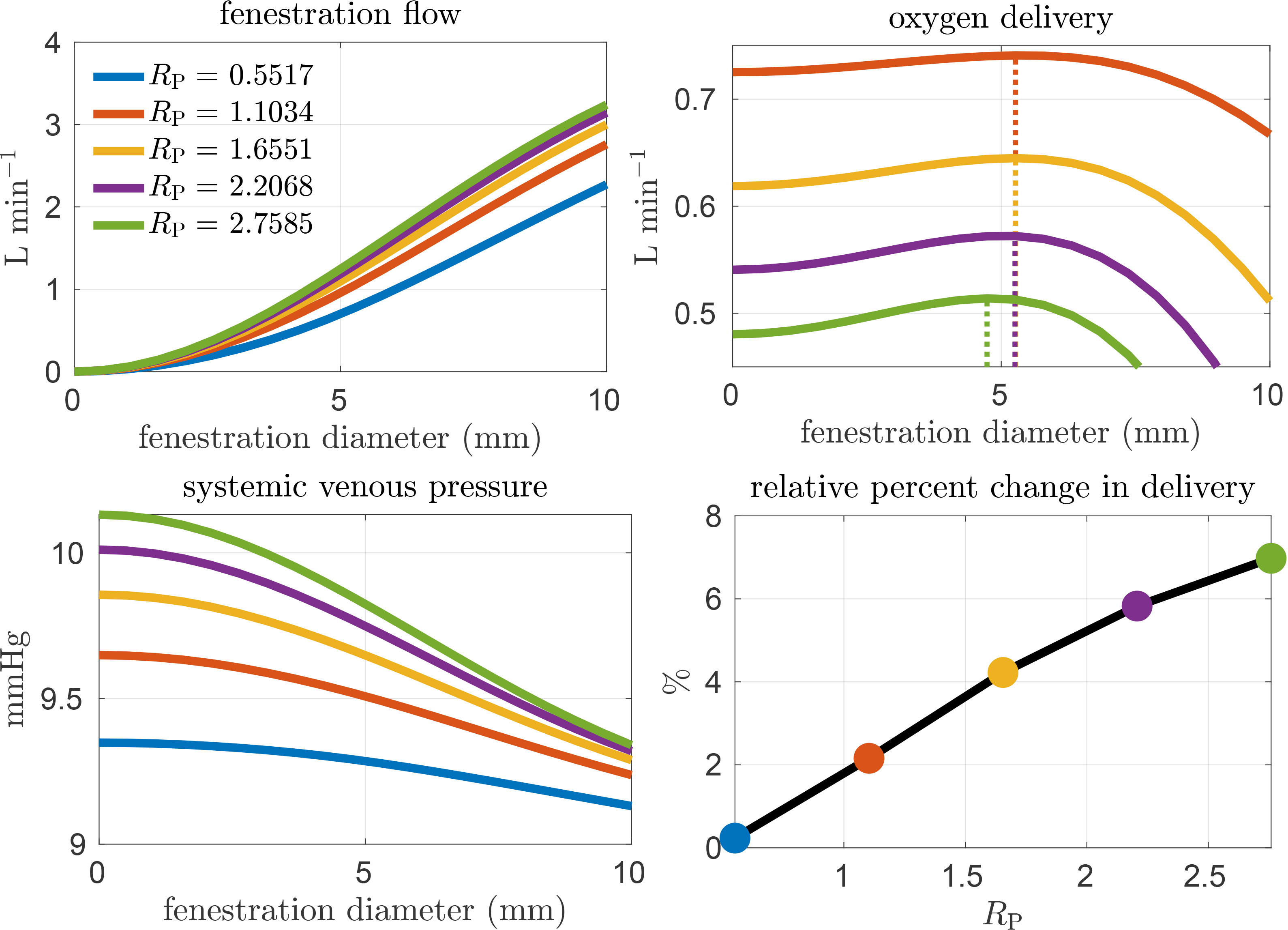
The circulation model enables the optimization of fenestration diameter to maximize oxygen delivery, thereby balancing the tradeoff between increased flow and decreased oxygen content. The results demonstrate that as the fenestration’s diameter increases, blood flow becomes greater and more pulsatile (see Figure 2). Furthermore, while oxygen saturation does decrease, oxygen delivery remains fairly constant up to a certain threshold of fenestration size. There is simultaneously a large reduction in systemic venous pressure. “The benefits from this procedure appear to be more substantial for high-risk patients,” Ahmad said. “For those who have a higher pulmonary resistance, or hypertension, there is a unique fenestration size that yields maximum oxygen delivery.”

Since heart rate is a fixed parameter, this model breaks down in response to dynamic situations such as exercise. Ahmad thus implemented a controller that responds to the reduction of systemic resistance at a certain time to signify the start of exercise. The improved control model detects the corresponding decrease in the filtered output of the arterial pressure and subsequently increases a new variable heart rate function. As the compliance function takes a fixed heart rate as input, solving a differential equation finds a mapping between the time in the simulation and the appropriate input time for the compliance function based on the new heart rate. Ahmad initially tried fixing the set pressure in the model to the mean arterial pressure, but the results were not ideal; however, increasing the set pressure at the start of exercise did lead the system to behave as expected.

Overall, the pulsatile model clearly indicates the benefits of fenestration for both low- and high-risk Fontan patients. “We also showed a simple method for varying heart rate that can be calibrated for circulation response to dynamic situations,” Ahmad said. In the future, he hopes to further model the impact of exercise on fenestrated Fontan circulation and investigate the effects of high acceleration.

Circulatory Response to Hypergravity

Kennard has worked with Ahmad, Peskin, and Karen Ong and Rebecca Blue (both of the University of Texas Medical Branch) to create a dynamic mathematical model of the human circulatory system that incorporates control to study the physiological response to hypergravity. Ahmad delivered the second talk of the CT23 minisymposium session, which overviewed these efforts, on Kennard’s behalf. Kennard later stated that when building a model, her focus lies primarily on utility. “If math modeling is going to be useful and make its way out of mathematician circles and into industry or decision-making, the model needs to be fast, easy to parametrize, and above all possessive of some predictive value,” she said.

Hypergravity—a much higher acceleration or gravitational pull than is usually encountered in everyday life on Earth—can alter blood flow and cause partial or total loss of consciousness. Astronauts and fighter pilots both experience this phenomenon and use various strategies to combat its symptoms. As a new era of space tourism commences and people without the extreme physical fitness of astronauts begin to explore space, researchers must answer two questions: how much gravitational pull can a particular individual tolerate, and at what point do the symptoms become overwhelming?

Kennard worked with Peskin—whose research on physiological modeling often incorporates optimal control—to develop a real-time dynamic model of the human circulatory system. She then used data from Ong and Blue’s ongoing centrifuge study to evaluate model response for real test subjects. “Basically, we spin subjects to simulate hypergravity and monitor their response,” Ahmad explained during the CT23 presentation.

Kennard had previously collaborated with Ahmad, Peskin, Ong, and Blue to create a steady-state control model that predicted individualized tolerance for hypergravity. This model was fast and easy to parametrize, but did not address the second question: when do subjects lose consciousness?

“How do we preserve the benefits of a fast model, while including the nonlinear effects of time dependency?” Ahmad asked. Most dynamic models of the circulatory system are pulsatile partial differential equation (PDE) models, which tend to be computationally expensive and difficult to calibrate. Pulsatile PDE models make intuitive sense, as they can depict a heartbeat as a pulse or waveform and represent the flow through arteries and veins as flow through a connected set of deformable cylinders. However, choosing the right granularity to calibrate such a model with readily measurable physiological data is a challenge — not to mention the issue of computational efficiency. Ong and Blue’s aerospace medical expertise became crucial for these modeling decisions. “It’s really helpful to know how feasible a measurement is,” Kennard later said.

The collaborators addressed these challenges by designing an ordinary differential equation model for the systemic circulation, a negative feedback controller to model baroreceptor behavior, and a continuous flow model of blood flow through the heart. This framework can ignore the mechanics of heart and lung compartments. “The outcomes that matter to us—drops in blood pressure and blood flow to the brain—happen in the body, not the heart,” Kennard said. 

Kennard’s model divides circulation into two types of compartments: compliance chambers and resistance elements. The arterial pressure is re-expressed in terms of state variables to obtain a linear system, in which the unknowns are the upper and lower arterial pressures. “Then, we want to model partial venous collapse, which is where external veins collapse before the heart,” Ahmad said. The model can take time-dependent parameters as input, like the vertical acceleration that subjects experienced in the centrifuge as well as their physiological measurements such as height and weight.

Ahmad presented several examples in which the model accurately depicted the change in heart rate, atrial pressure, and systemic volume in response to hypergravity. To cap off the minisymposium talk, he summarized that the non-pulsatile model is able to effectively model physiologically reasonable behavior under hypergravity, but that more test subjects are required to properly evaluate the model’s predictive value. Moving forward, Kennard intends to apply the model to a larger population from the centrifuge study to validate its hypergravity tolerance predictability. 

References

[1] Ahmad, Z., Jin, L.H., Penny, D.J., Rusin, C.G., Peskin, C.S., & Puelz, C. (2022). Optimal fenestration of the Fontan circulation. Front. Physiol., 13, 867995.