Further Upon Reflection: Caustics in a Ball

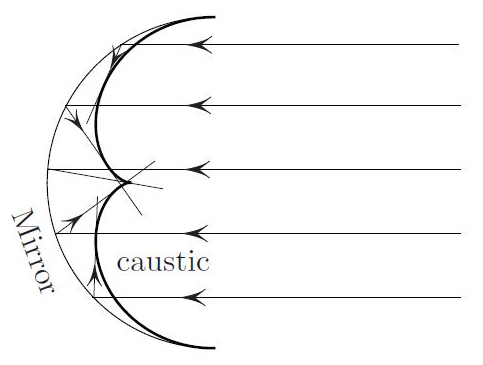
What can one say about the location of the sun’s reflection in a shiny ball? Before discussing the answer, let us first consider a circular mirror with a reflecting inner (rather than outer) surface (see Figure 1), illuminated by a pencil of incoming parallel rays — like the top view of a cup in the sun. The bright line on the bottom of the cup is the envelope of the family of reflected rays; such envelopes are called caustics.

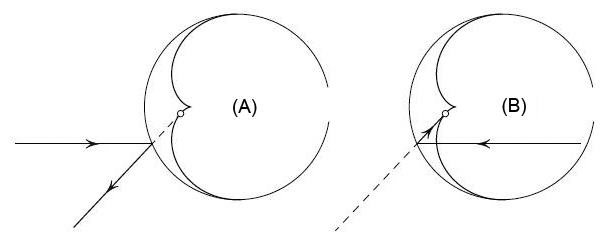
Interestingly, the exact same caustic is the locus of the sun’s reflections from the outside surface of the same circular mirror (see Figure 2). The caustic in Figure 2 is the envelope of (the lines defined by) reflected rays. Since the same words describe the caustic in Figure 1, the two caustics are indeed identical; Figure 3 depicts the outer and inner reflections side-by-side, illustrating the identity of the two caustics in one picture.

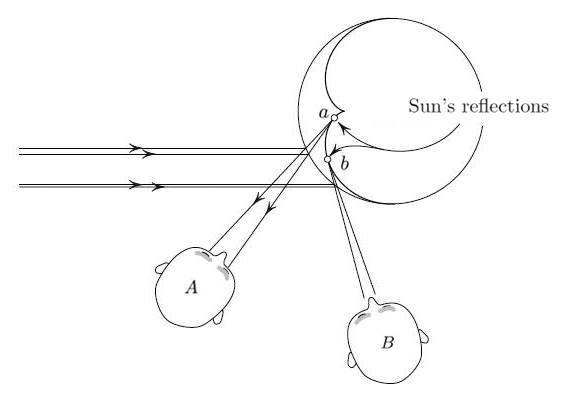
Now, why do the sun’s reflections lie on the caustic? Figure 2 provides the answer: infinitesimally speaking, each point (such as \(a\) or \(b\)) on the caustic/envelope acts as the point source of light. To the observer \(A\), it will thus appear as if a point source of light exists at \(a\). To summarize, every reflection in any shiny mirror lies on a caustic, i.e., on the envelope of reflected rays. This holds true for a mirror of any shape, not only a circle.

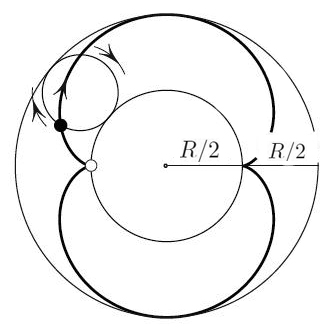
For the circular mirrors in Figures 1 and 2, the caustic happens to be a nephroid, i.e., a hypercycloid traced by a point on the rolling wheel, as shown in Figure 4. I provided a short proof of this fact in my April column.

The figures in this article were provided by the author.