Minimizing the Length — and the Algebra

With the semester in full swing and some SIAM News readers likely teaching calculus, I thought of an amusing solution to the standard problem of minimizing the length of a fence that encloses three sides of a rectangular pasture of fixed area (the remaining side is bordered by a wall).

The Analog Computer

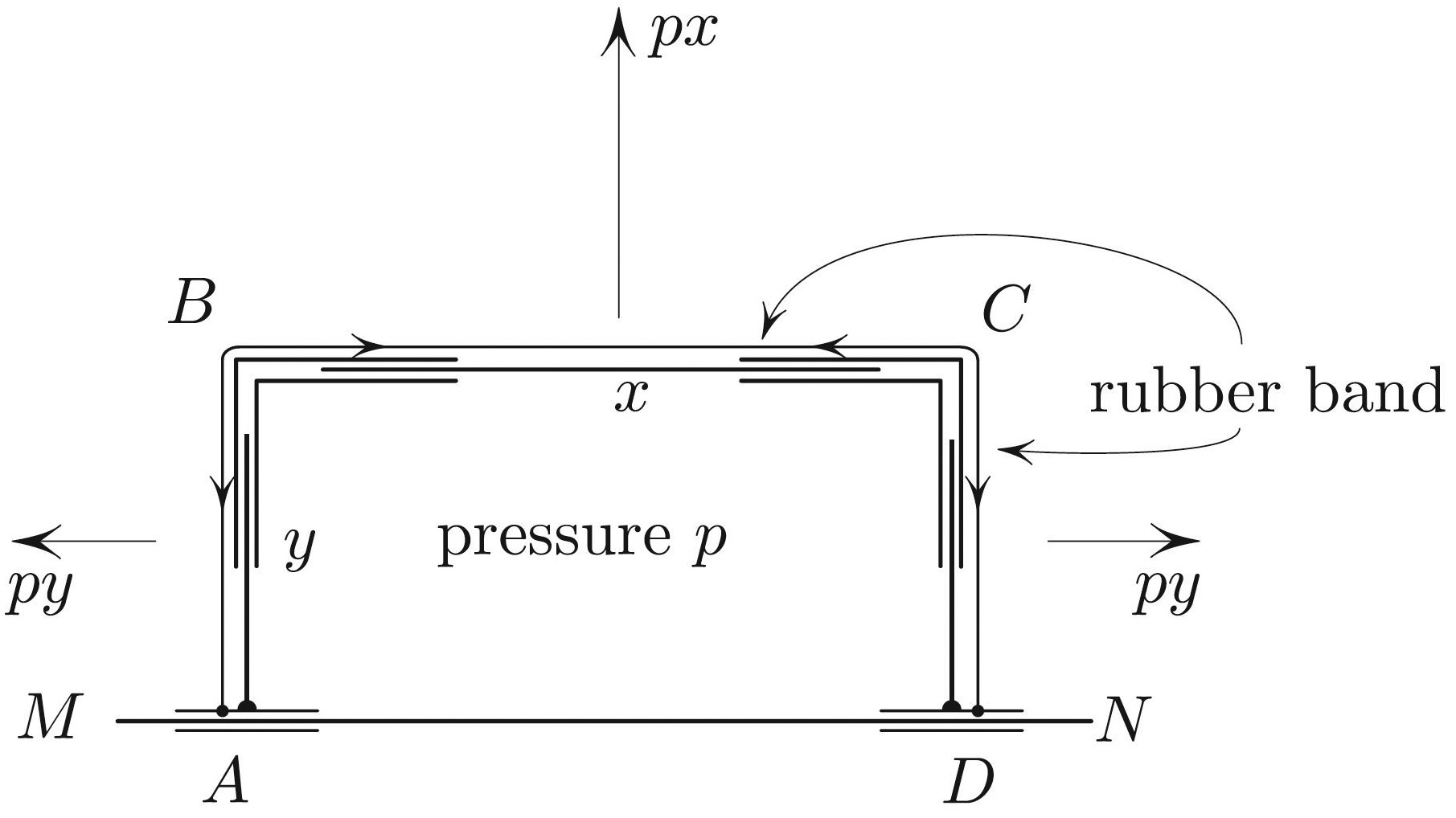
Figure 1 shows a mechanical “analog computer” from which one can simply read off a solution. The telescoping rectangle encloses incompressible two-dimensional fluid; the area is thus fixed. Sliders \(A\) and \(D\) can move without friction along the “wall” \(MN\). All telescoping connections are frictionless, and a slippery rubber band is wrapped around the frame and affixed to sliders at \(A\) and \(D\). Since the rubber band is slippery, its tension \(T\) is the same throughout its length.

The Solution

The band tries to minimize the length, and the minimal length shape is in equilibrium. But then the vertical forces acting on the top side of the rectangle are in balance (see Figure 2): \[px=2T.\tag1\] Similarly, the left-right forces on a vertical side, say \(CD\), are in balance as well: \[py=T.\tag2\] Thus, \(x=2y\) and the problem is solved; the ratio of the optimal rectangle’s sides is \(1:2\). 

Discussion

It is interesting to compare this admittedly nonrigorous solution to the textbook treatment in first-semester calculus. In our “solution,” I replaced algebraic manipulation and differentiation with the work of inventing a mechanical device. I only meant this as a perhaps amusing complement to the “standard approach,” rather than as a replacement. Incidentally, the vanishing of the perimeter’s derivative (with respect to one of the lengths) is equivalent to stating that the tension of the rubber band is the same along all three walls.

The figures in this article were provided by the author.