The Mathematics of Poverty, Inequality, and Oligarchy

In 2019, Oxfam reported that the 26 richest people in the world held as much wealth as the poorest half of the global population [9]. Such staggering levels of inequality have precipitated much interdisciplinary discussion among economists, political scientists, sociologists, urban planners, and ethicists. Here, we outline some recent mathematical work on this subject. Our approach applies methods from the kinetic theory of gases to simplified models of the economy in order to explore both potential mechanisms by which wealth inequality arises and the effects of government interventions that attempt to reduce it.

Pareto Tails, Gini Coefficients, and Lorenz Curves

In the second half of the 19th century, Italian polymath Vilfredo Pareto found that the distribution of wealth of a randomly selected individual is often well approximated by a continuous distribution with a heavy, algebraic tail:

\[1- F(w) \sim C w^{-\alpha} \enspace \textrm{as} \enspace w \rightarrow \infty.\]

Fits to empirical data suggest that \(1 < \alpha < 2\) in most Western industrialized countries, including the U.S. [11]. The mean \(\mu\) of \(F\) is therefore finite, but its variance is infinite; wealth variance is hence not a useful measure of wealth inequality. A well-known alternative is the Gini coefficient \(G\), which Italian statistician Corrado Gini proposed in 1912. If \(V\) and \(W\) are the wealths of two individuals who are independently chosen at random from the population, then

\[G = \frac{E(|W-V|)}{2 \mu}.\tag1\]

Finiteness of \(G\) does not require the existence of moments of \(F\) of order greater than \(1\). Clearly \(G \geq 0\), and \(G \leq 1\) by the triangle inequality. There is no distribution on \([0,\infty)\) with \(G=1\), but some distributions come arbitrarily close. For instance, if \(\epsilon \in (0,1)\), then the distribution with generalized density

\[(1-\epsilon) \delta(w) + \epsilon \delta \left( w - \frac{\mu}{\epsilon} \right)\tag2\]

has \(G=1-\epsilon\).

In 1905, American economist Max Lorenz defined a function \(L\) on \([0,1]\) by

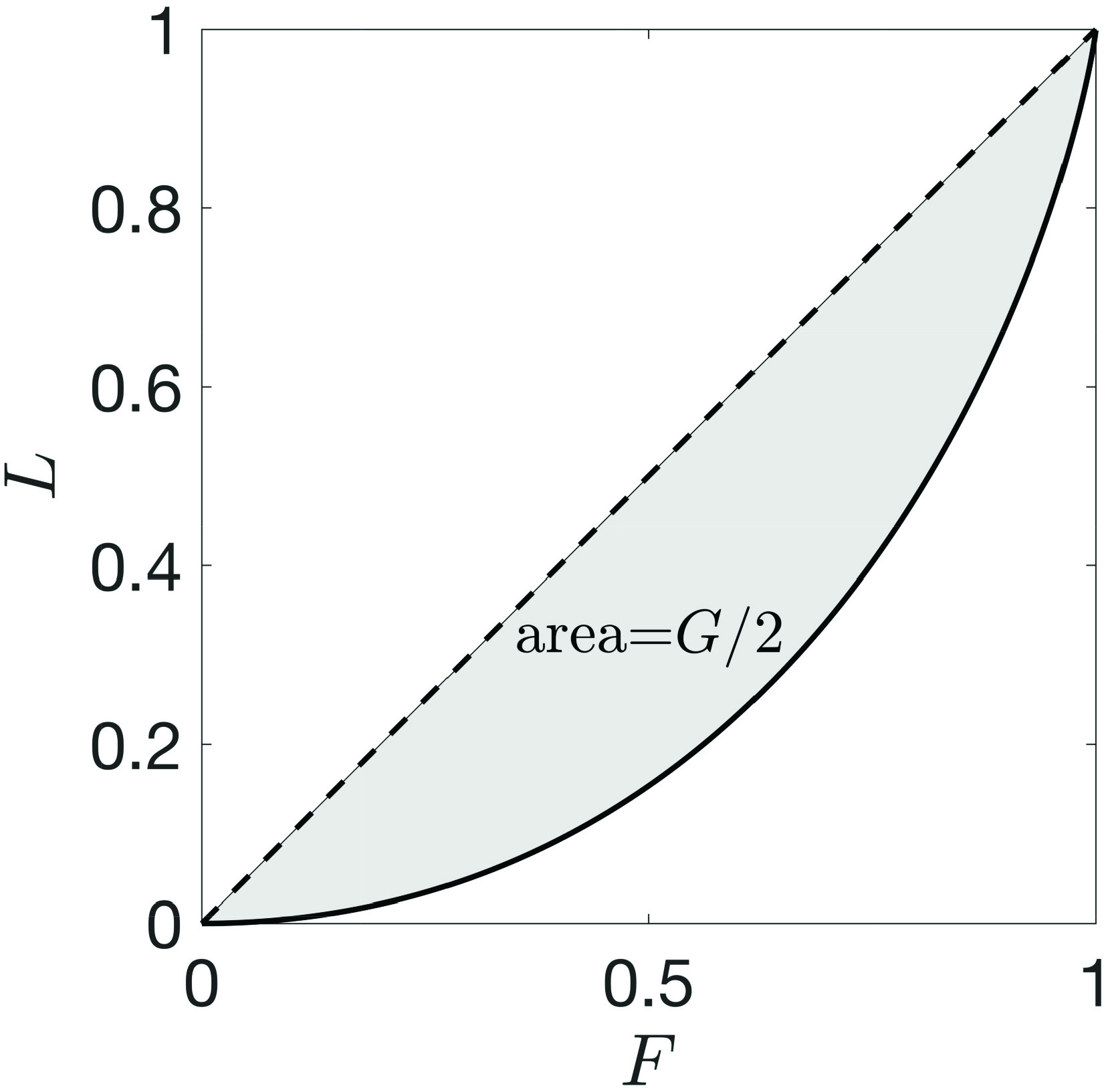
\[L(F(w)) = \frac{E(W \cdot 1_{W \leq w} )}{\mu}\]

(this formula defines a function on \([0,1]\) if \(F\) is continuous). Its graph is called the Lorenz curve. We have \(L(0)=0\) and \(L(1)=1\), and \(L\) is increasing and convex. Verifying the well-known geometric interpretation of \(G\) in Figure 1 is an exercise in calculus.

Yard-sale Model

To understand the emergence of wealth distributions, one must study the dynamics of wealth. In 2002, Anirban Chakraborti drew from the microscopic theory of gases to propose the yard-sale model of an economy [7]. There are \(N\) agents in this model. In each time step, a random pair of agents interacts. When an interaction occurs between agents \(j\) and \(k\) and their pre-interaction wealths are \(w_j\) and \(w_k\), the transaction causes a wealth transfer of size

\[\beta~ \min(w_j, w_k),\tag3\]

where \(\beta>0\) is small. The transfer of wealth takes place because people make mistakes and over- or underpay due to imperfect information. The amount at stake in each transaction is a small fraction of the poorer agent’s wealth because people do not usually enter transactions that jeopardize a large fraction of their total assets. A fair coin flip determines the direction of wealth transfer.

Though all seems fair, computer simulations reveal that the most extreme form of oligarchy invariably emerges; one agent eventually owns the entire economy. This phenomenon is called wealth condensation [6]. Most or all of a poor agent’s transactions change the agent’s wealth multiplicatively, by a factor of \(1 \pm \beta\). Since the number of unfavorable versus favorable transactions is roughly the same, one can think of the transactions in pairs. Each pair changes the agent’s wealth by

\[(1+\beta)(1-\beta) = 1- \beta^2 < 1,\tag4\]

meaning that a poor agent loses wealth over time. Because the model conserves wealth, somebody else must gain. The conclusion is utterly striking: free and fair trading among identical agents inescapably results in extreme wealth inequality.

This phenomenon was studied in a version of the model with a continuum of agents and continuous time [4]. Between agents of wealth \(w\) and \(x\), we assume that there is a flow of wealth in time \(\Delta t\) of magnitude

\[\sqrt{\gamma \Delta t} \min(w,x),\tag5\]

where \(\gamma>0\) is a reciprocal time. The factor \(\sqrt{\gamma\Delta t}\) in \((5)\) plays the role of \(\beta\) in \((3)\). The direction of the flow of wealth is random and unbiased, which implies that the wealth change of an agent with wealth \(w\) on account of an agent with wealth \(x\) in time \(\Delta t\) has a mean \(0\) and variance \(\gamma \Delta t \min(w,x)^2\). The wealth density \(f=f(w,t)\) therefore satisfies the following nonlinear, nonlocal Fokker-Planck equation [1, 2]:

\[\frac{\partial f}{\partial t} (w,t) = \frac{ \gamma}{2} \left( \left( \int_0^\infty (\min(x,w))^2 ~\! f(x,t) ~\! dx \right) f(w,t) \right)_{ww}.\tag6\]

The minimum \(\min(x,w)\) appears because the amount at stake in a single transaction is proportional to the poorer agent’s wealth.

Christophe Chorro observed that from a probabilistic point of view, convergence to total oligarchy in the yard-sale model is an almost immediate consequence of the martingale convergence theorem [8]. For a more elementary probabilistic proof, which also allows coin flips that are biased in favor of the wealthier agent, see [5].

The Extended Yard-sale Model

Over the years, various effects have been added to the yard-sale model to enhance its realism, such as wealth redistribution [1, 2]. We assume that the government taxes each agent a fraction \(\gamma\chi\) of their wealth per unit time and redistributes it to all agents in equal shares. Doing so adds a simple linear advection term to the Fokker-Planck equation—the first advection term in \((7)\)—and drives each agent’s wealth towards the mean \(\mu\). This new term prevents wealth condensation and yields reasonably realistic wealth distributions [10].

Another addition is a wealth-acquired advantage [3], which reflects the fact that wealthy individuals can afford better information, better lawyers, more effective lobbyists, and so forth. We again assume that \((5)\) describes the magnitude of the wealth shift between agents of wealth \(w\) and \(x\) in time \(\Delta t\), but now we take the direction of wealth flow as slightly biased in favor of the wealthier agent. The probability of a shift from \(x\) to \(w\) (rather than the other way around) is

\[\frac{1}{2} \left( 1 + \zeta \sqrt{\gamma \Delta t} ~\! \frac{w-x}{\mu} \right).\]

Here, \(\zeta>0\) is a new nondimensional model parameter. The expected total flow of wealth from \(x\) to \(w\) in time \(\Delta t\) is now 

\[\gamma \zeta \min(x,w) \frac{w-x}{\mu} \Delta t,\]

which gives rise to the second nonlinear and nonlocal advection term in \((7)\). The Fokker-Planck equation for the extended yard-sale model thus becomes

\[\frac{\partial f}{\partial t} (w,t) + \gamma \chi \left( (\mu - w) f(w,t) \right)_w + \gamma \zeta \left( \left( \int_0^\infty \min(x,w) \frac{w-x}{\mu} f(x,t) dx \right) f(w,t) \right)_w =\]

\[\\ \frac{\gamma}{2} \left(\left(\int_0^\infty (\min(x,w))^2 f(x,t) dx \right) f(w,t) \right)_{ww}.\tag7\]

In the next section, we will show that \((7)\) implies the return of partial oligarchy if the wealth-acquired advantage is strong enough, or if wealth taxation is too weak.

Oligarchy

To model situations in which a vanishingly small fraction of the population holds a positive fraction of society’s wealth, we define a new distribution on \([0,\infty)\)—denoted by \(\Xi\)—with total mass \(0\) but expectation \(1\):

\[\langle \Xi, 1 \rangle = 0, ~~~ \langle \Xi , w \rangle = 1.\]

This distribution was constructed in [2] by augmenting the Schwartz test functions with functions that have linear growth as \(w \rightarrow \infty\), defining \(\langle \Xi, \varphi \rangle = \textrm{lim}_{w \rightarrow \infty} \frac{\varphi(w)}{w}\) or equivalently

\[\langle \Xi, \varphi \rangle = \lim_{\epsilon \rightarrow 0} \left \langle \epsilon \delta \left( w - \frac{1}{\epsilon} \right), \varphi \right \rangle.\]

Because the (generalized) density \((2)\) has Gini coefficient \(1-\epsilon\), we might say that \(\delta(w) + \mu \Xi(w)\) has Gini coefficient \(1\) and represents total oligarchy — with a vanishingly small fraction owning everything and all others owning nothing.

In [3], it was shown that \((7)\) admits solutions of the form

\[f(w,t) = c(t) \mu \Xi(w) + \rho(w,t). \]

The term \(c(t) \mu \Xi(w)\) represents a fraction \(c(t)\) of society’s wealth that is held by a vanishingly small number of people, henceforth called the oligarchs. The term \(\rho(w,t)\) is a probability density of mean \((1-c(t)) \mu\) that represents the wealth distribution among everyone who is not an oligarch. The function \(c(t)\) satisfies the logistic equation \[\frac{dc}{dt} = - \gamma~\! \chi ~\! c +\gamma~\! \zeta ~\! c (1-c). \tag8\] The term \(- \gamma \chi c\) reflects the oligarchs’ loss of wealth due to wealth taxation, and \(\gamma \zeta c (1-c)\) reflects the growth of the oligarchs’ wealth on account of their wealth-acquired advantage. Equation \((8)\) has two fixed points: \(c=0\) and \(c=1 - \frac{\chi}{\zeta}\). For \(\zeta<\chi\), \(c=0\) is a stable fixed point and \(c\)—starting at a non-negative value—will converge to \(0\); we call this the subcritical regime. For \(\zeta>\chi\), \(c=0\) is an unstable fixed point and \(c\)—again starting at a non-negative value—will converge to \(1 - \frac{\chi}{\zeta}>0\); we call this the supercritical regime. The transition from one regime to the other is a transcritical bifurcation. 

In 2016, the ratio \(\zeta/\chi\) for the U.S. economy was estimated at about \(1.39\), suggesting that the U.S. was well in the supercritical (oligarchic) regime [10].

Comparison with Empirical Results

While the extended yard-sale model originally served as a rough qualitative model of wealth distribution and oligarchy, a slight modification that allows for agents of negative wealth is remarkably faithful to empirical wealth data. This modification rigidly shifts the probability density function in the direction of negative wealth by an amount \(\kappa\mu\), where \(\kappa\) is a new parameter. The resulting three-parameter \((\chi,\zeta,\kappa)\) affine wealth model admits steady-state solutions whose Lorenz curves match those of empirical wealth distributions to within one fifth of one percent for American wealth data between 1987 and 2019, and to within a half of a percent for 14 European countries that were associated with the European Central Bank circa 2010. This is far more accurate than fits to Pareto distributions; in fact, it provides perhaps the most accurate microfounded model of wealth distribution to date.

While surely not the last word on models of wealth distribution, the extended yard-sale model and affine wealth model demonstrate the utility and effectiveness of kinetic theory in modern efforts to understand and quantify the origins and time evolution of economic inequality. Despite being at odds with orthodox neoclassical economics—for example, the notion that trade is always deterministic and rational and consistently benefits both participating agents—these models can very accurately explain empirical wealth distributions, including the phenomenon of oligarchy. 

References 

[1] Boghosian, B.M. (2014). Fokker-Planck description of wealth dynamics and the origin of Pareto’s law. Int. J. Mod. Phys. C, 25(12), 1441008. 
[2] Boghosian, B.M. (2014). Kinetics of wealth and the Pareto law. Phys. Rev. E, 89, 042804.
[3] Boghosian, B.M., Devitt-Lee, A., Johnson, M., Li, J., Marcq, J.A., & Wang, H. (2017). Oligarchy as a phase transition: The effect of wealth-attained advantage in a Fokker-Planck description of asset exchange. Physica A: Stat. Mech. Appl., 476, 15-37.
[4] Boghosian, B.M., Johnson, M., & Marcq, J.A. (2015). An H theorem for Boltzmann’s equation for the yard-sale model of asset exchange. J. Stat. Phys., 16, 1339-1350.
[5] Börgers, C., & Greengard, C. (2023). A new probabilistic analysis of the yard-sale model. Preprint, arXiv:2308.01485.
[6] Bouchaud, J.-P., & Mézard, M. (2000). Wealth condensation in a simple model of economy. Physica A: Stat. Mech. Appl., 282(3-4), 536-545.
[7] Chakraborti, A. (2002). Distributions of money in model markets of economy. Int. J. Mod. Phys. C, 13(10), 1315-1321.
[8] Chorro, C. (2016). A simple probabilistic approach of the yard-sale model. Stat. Prob. Lett., 112, 35-40.
[9] Lawson, M., Chan, M.-K., Rhodes, F., Butt, A.P., Marriott, A., Ehmke, E., … Dorling, D. (2019). Public good or private wealth? (Oxfam briefing paper – January 2019). Oxford, UK: Oxfam International.
[10] Li, J., Boghosian, B.M., & Li, C. (2019). The affine wealth model: An agent-based model of asset exchange that allows for negative-wealth agents and its empirical validation. Physica A: Stat. Mech. Appl., 516, 423-442.
[11] Vermeulen, P. (2015). How fat is the top tail of the wealth distribution? (Working paper series no. 1692). Household Finance and Consumption Network: European Central Bank.